October 2021 – Page 3 – My Programming Notes

Monthly Archives: October 2021

C# || How To Determine If Binary Tree Root To Leaf Path Sum Exists Using C#

October 18, 2021 admin No comments

The following is a module with functions which demonstrates how to determine if a binary tree root to leaf path sum exists using C#.

1. Has Path Sum – Problem Statement

Given the root of a binary tree and an integer targetSum, return true if the tree has a root-to-leaf path such that adding up all the values along the path equals targetSum.

A leaf is a node with no children.

Example 1:

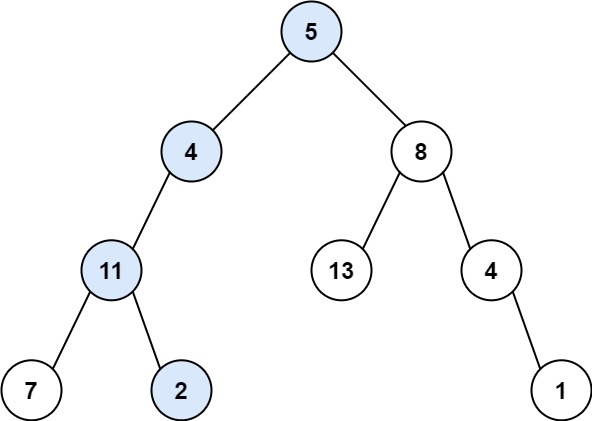
Input: root = [5,4,8,11,null,13,4,7,2,null,null,null,1], targetSum = 22 Output: true

Example 2:

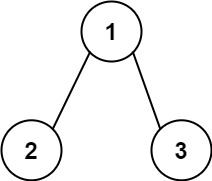
Input: root = [1,2,3], targetSum = 5 Output: false

Example 3:

Input: root = [1,2], targetSum = 0 Output: false

2. Has Path Sum – Solution

The following is a solution which demonstrates how to determine if a binary tree root to leaf path sum exists.





		
		
			2. Has Path Sum - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 18, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines if a root to leaf path sum exists in a binary tree
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    public bool HasPathSum(TreeNode root, int targetSum) {
        return Search(root, targetSum);
    }

    public bool Search(TreeNode node, int targetSum) {
        if (node == null) {
            return false;
        }

        // Determine the current sum
        targetSum = targetSum - node.val;

        // Since this is a root-to-leaf check, evaluate for the
        // success condition when both left and right nodes are null
        if (node.left == null && node.right == null) {
            return targetSum == 0;
        }

        // Keep exploring along branches finding the target sum
        return Search(node.left, targetSum)
            || Search(node.right, targetSum);
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 18, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines if a root to leaf path sum exists in a binary tree
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    public bool HasPathSum(TreeNode root, int targetSum) {
        return Search(root, targetSum);
    }
 
    public bool Search(TreeNode node, int targetSum) {
        if (node == null) {
            return false;
        }
 
        // Determine the current sum
        targetSum = targetSum - node.val;
 
        // Since this is a root-to-leaf check, evaluate for the
        // success condition when both left and right nodes are null
        if (node.left == null && node.right == null) {
            return targetSum == 0;
        }
 
        // Keep exploring along branches finding the target sum
        return Search(node.left, targetSum)
            || Search(node.right, targetSum);
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

true false false

C# || How To Convert Sorted Array To Binary Search Tree Using C#

October 18, 2021 admin No comments

The following is a module with functions which demonstrates how to convert a sorted array to a binary search tree using C#.

1. Sorted Array To BST – Problem Statement

Given an integer array nums where the elements are sorted in ascending order, convert it to a height-balanced binary search tree.

A height-balanced binary tree is a binary tree in which the depth of the two subtrees of every node never differs by more than one.

Example 1:

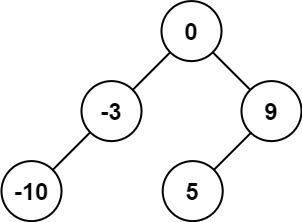
Input: nums = [-10,-3,0,5,9] Output: [0,-3,9,-10,null,5] Explanation: [0,-10,5,null,-3,null,9] is also accepted:

Example 2:

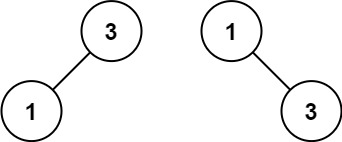
Input: nums = [1,3] Output: [3,1] Explanation: [1,3] and [3,1] are both a height-balanced BSTs.

2. Sorted Array To BST – Solution

The following is a solution which demonstrates how to convert a sorted array to a binary search tree.





		
		
			2. Sorted Array To BST - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 18, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to convert sorted array to BST
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    public TreeNode SortedArrayToBST(int[] nums) {
        return BuildBST(nums, 0, nums.Length -1);
    }

    TreeNode BuildBST(int[] nums, int start, int end) {
        if (start > end) {
            return null;
        }

        var mid = start + (end - start) / 2;
        var node = new TreeNode(nums[mid]);
        node.left = BuildBST(nums, start, mid - 1);
        node.right = BuildBST(nums, mid + 1, end);
        return node;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 18, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to convert sorted array to BST
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    public TreeNode SortedArrayToBST(int[] nums) {
        return BuildBST(nums, 0, nums.Length -1);
    }
 
    TreeNode BuildBST(int[] nums, int start, int end) {
        if (start > end) {
            return null;
        }
 
        var mid = start + (end - start) / 2;
        var node = new TreeNode(nums[mid]);
        node.left = BuildBST(nums, start, mid - 1);
        node.right = BuildBST(nums, mid + 1, end);
        return node;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[0,-10,5,null,-3,null,9] [1,null,3]

C# || How To Convert 1D Array Into 2D Array Using C#

October 18, 2021 admin No comments

The following is a module with functions which demonstrates how to convert a 1D array into a 2D array using C#.

1. Construct 2D Array – Problem Statement

You are given a 0-indexed 1-dimensional (1D) integer array original, and two integers, m and n. You are tasked with creating a 2-dimensional (2D) array with m rows and n columns using all the elements from original.

The elements from indices 0 to n – 1 (inclusive) of original should form the first row of the constructed 2D array, the elements from indices n to 2 * n – 1 (inclusive) should form the second row of the constructed 2D array, and so on.

Return an m x n 2D array constructed according to the above procedure, or an empty 2D array if it is impossible.

Example 1:

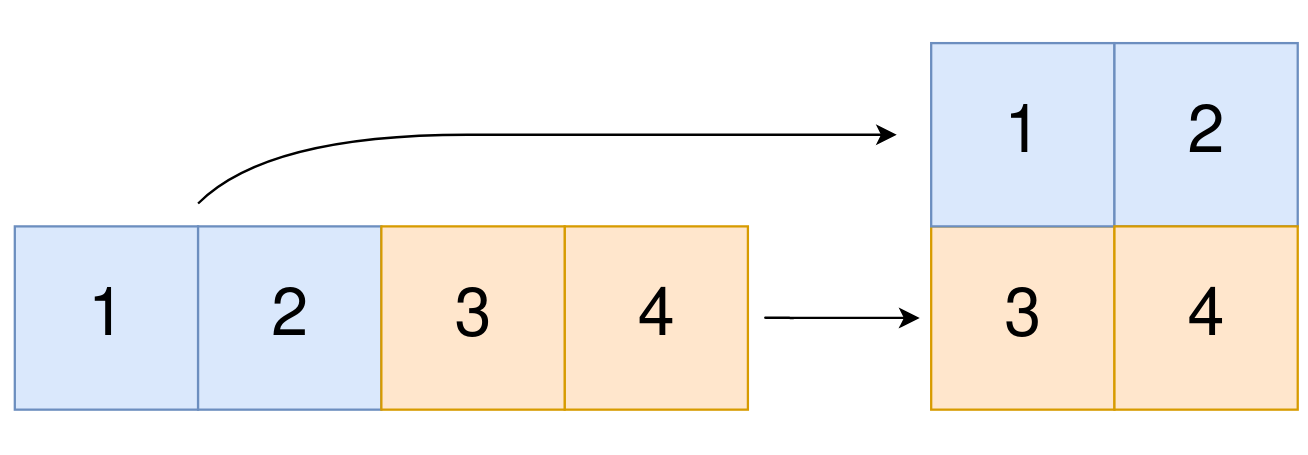
Input: original = [1,2,3,4], m = 2, n = 2 Output: [[1,2],[3,4]] Explanation: The constructed 2D array should contain 2 rows and 2 columns. The first group of n=2 elements in original, [1,2], becomes the first row in the constructed 2D array. The second group of n=2 elements in original, [3,4], becomes the second row in the constructed 2D array.

Example 2:

Input: original = [1,2,3], m = 1, n = 3 Output: [[1,2,3]] Explanation: The constructed 2D array should contain 1 row and 3 columns. Put all three elements in original into the first row of the constructed 2D array.

Example 3:

Input: original = [1,2], m = 1, n = 1 Output: [] Explanation: There are 2 elements in original. It is impossible to fit 2 elements in a 1x1 2D array, so return an empty 2D array.

Example 4:

Input: original = [3], m = 1, n = 2 Output: [] Explanation: There is 1 element in original. It is impossible to make 1 element fill all the spots in a 1x2 2D array, so return an empty 2D array.

2. Construct 2D Array – Solution

The following is a solution which demonstrates how to convert a 1D array into a 2D array.





		
		
			2. Construct 2D Array - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 18, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to convert a 1D array into a 2D array
// ============================================================================
public class Solution {
    public int[][] Construct2DArray(int[] original, int m, int n) {
        // Determine if parameters are valid
        if (original.Length != m * n) {
            return new int[0][];
        }

        // Create 2D array
        var result = new int[m][];
        for (int row = 0; row < result.Length; ++row) {
            result[row] = new int[n];
        }

        // Populate result
        for (int index = 0; index < original.Length; ++index) {
            // Determine the result array row and column index
            var rowIndex = index / n;
            var colIndex = index % n;

            // Copy values to result
            result[rowIndex][colIndex] = original[index];
        }

        return result;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 18, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to convert a 1D array into a 2D array
// ============================================================================
public class Solution {
    public int[][] Construct2DArray(int[] original, int m, int n) {
        // Determine if parameters are valid
        if (original.Length != m * n) {
            return new int[0][];
        }
 
        // Create 2D array
        var result = new int[m][];
        for (int row = 0; row < result.Length; ++row) {
            result[row] = new int[n];
        }
 
        // Populate result
        for (int index = 0; index < original.Length; ++index) {
            // Determine the result array row and column index
            var rowIndex = index / n;
            var colIndex = index % n;
 
            // Copy values to result
            result[rowIndex][colIndex] = original[index];
        }
 
        return result;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[[1,2],[3,4]] [[1,2,3]] [] []

C# || How To Find Cousins In A Binary Tree Using C#

October 17, 2021 admin No comments

The following is a module with functions which demonstrates how to find the cousins in a binary tree using C#.

1. Is Cousins – Problem Statement

Given the root of a binary tree with unique values and the values of two different nodes of the tree x and y, return true if the nodes corresponding to the values x and y in the tree are cousins, or false otherwise.

Two nodes of a binary tree are cousins if they have the same depth with different parents.

Note that in a binary tree, the root node is at the depth 0, and children of each depth k node are at the depth k + 1.

Example 1:

Input: root = [1,2,3,4], x = 4, y = 3 Output: false

Example 2:

Input: root = [1,2,3,null,4,null,5], x = 5, y = 4 Output: true

Example 3:

Input: root = [1,2,3,null,4], x = 2, y = 3 Output: false

2. Is Cousins – Solution

The following is a solution which demonstrates how to determine if x and y values are cousins in a binary tree.

The idea here is to explore each path, finding the node values that represent x and y.

Once both values are found, save the depth and parent node, and determine if they are cousins





		
		
			2. Is Cousins - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 17, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines if x and y are cousins in a binary tree
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    // For the x parent and depth values
    private int xParent = -1;
    private int xDepth = -1;

    // For the y parent and depth values
    private int yParent = -1;
    private int yDepth = -1;

    public bool IsCousins(TreeNode root, int x, int y) {
        Search(root, x, y, root.val, 0);

        // Check for success condition
        return xParent != yParent && xDepth == yDepth;
    }

    private void Search(TreeNode node, int x, int y, int parent, int depth) {
        // Values have been found
        if (xParent != -1 && yParent != -1) {
            return;
        }

        if (node == null) {
            return;
        }

        if (node.val == x) {
            // Set the x parent and depth values
            xParent = parent;
            xDepth = depth;
        } else if (node.val == y) {
            // Set the y parent and depth values
            yParent = parent;
            yDepth = depth;
        } else {
            // Keep exploring along branches for the success condition
            Search(node.left, x, y, node.val, depth + 1);
            Search(node.right, x, y, node.val, depth + 1);
        }
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 17, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines if x and y are cousins in a binary tree
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    // For the x parent and depth values
    private int xParent = -1;
    private int xDepth = -1;
 
    // For the y parent and depth values
    private int yParent = -1;
    private int yDepth = -1;
 
    public bool IsCousins(TreeNode root, int x, int y) {
        Search(root, x, y, root.val, 0);
 
        // Check for success condition
        return xParent != yParent && xDepth == yDepth;
    }
 
    private void Search(TreeNode node, int x, int y, int parent, int depth) {
        // Values have been found
        if (xParent != -1 && yParent != -1) {
            return;
        }
 
        if (node == null) {
            return;
        }
 
        if (node.val == x) {
            // Set the x parent and depth values
            xParent = parent;
            xDepth = depth;
        } else if (node.val == y) {
            // Set the y parent and depth values
            yParent = parent;
            yDepth = depth;
        } else {
            // Keep exploring along branches for the success condition
            Search(node.left, x, y, node.val, depth + 1);
            Search(node.right, x, y, node.val, depth + 1);
        }
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

false true false

C# || How To Construct A Binary Search Tree From Preorder Traversal Using C#

October 16, 2021 admin No comments

The following is a module with functions which demonstrates how to construct a binary search tree from preorder traversal using C#.

1. Binary Tree From Preorder – Problem Statement

Given an array of integers preorder, which represents the preorder traversal of a BST (i.e., binary search tree), construct the tree and return its root.

It is guaranteed that there is always possible to find a binary search tree with the given requirements for the given test cases.

A binary search tree is a binary tree where for every node, any descendant of Node.left has a value strictly less than Node.val, and any descendant of Node.right has a value strictly greater than Node.val.

A preorder traversal of a binary tree displays the value of the node first, then traverses Node.left, then traverses Node.right.

Example 1:

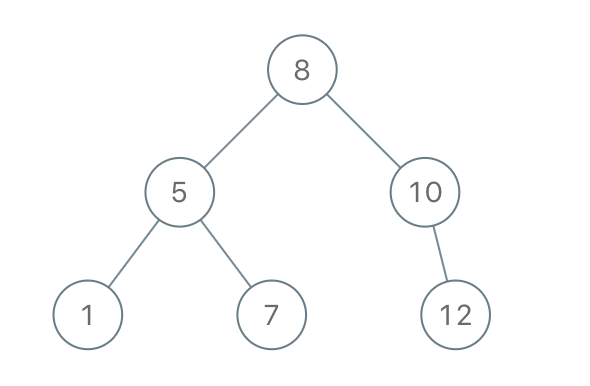
Input: preorder = [8,5,1,7,10,12] Output: [8,5,10,1,7,null,12]

Example 2:

Input: preorder = [1,3] Output: [1,null,3]

2. Binary Tree From Preorder – Solution

The following is a solution which demonstrates how to construct a binary search tree from preorder traversal.





		
		
			2. Binary Tree From Preorder - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 16, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to construct a BST from preorder traversal
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private int currentIndex = 0;

    public TreeNode BstFromPreorder(int[] preorder) {
        return BuildBST(preorder, int.MaxValue);
    }

    TreeNode BuildBST(int[] preorder, int parentValue) {
        if (currentIndex == preorder.Length || preorder[currentIndex] > parentValue) {
            return null;
        }

        var currentValue = preorder[currentIndex++];
        var node = new TreeNode(currentValue);
        node.left = BuildBST(preorder, currentValue);
        node.right = BuildBST(preorder, parentValue);
        return node;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 16, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to construct a BST from preorder traversal
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private int currentIndex = 0;
 
    public TreeNode BstFromPreorder(int[] preorder) {
        return BuildBST(preorder, int.MaxValue);
    }
 
    TreeNode BuildBST(int[] preorder, int parentValue) {
        if (currentIndex == preorder.Length || preorder[currentIndex] > parentValue) {
            return null;
        }
 
        var currentValue = preorder[currentIndex++];
        var node = new TreeNode(currentValue);
        node.left = BuildBST(preorder, currentValue);
        node.right = BuildBST(preorder, parentValue);
        return node;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[8,5,10,1,7,null,12] [1,null,3]

C# || How To Find The Next Greater Element In A Circular Array Using C#

October 15, 2021 admin No comments

The following is a module with functions which demonstrates how to find the next greater element in a circular array using C#.

1. Circular Next Greater – Problem Statement

Given a circular integer array nums (i.e., the next element of nums[nums.length – 1] is nums[0]), return the next greater number for every element in nums.

The next greater number of a number x is the first greater number to its traversing-order next in the array, which means you could search circularly to find its next greater number. If it doesn’t exist, return -1 for this number.

Example 1:

Input: nums = [1,2,1] Output: [2,-1,2] Explanation: The first 1's next greater number is 2; The number 2 can't find next greater number. The second 1's next greater number needs to search circularly, which is also 2.

Example 2:

Input: nums = [1,2,3,4,3] Output: [2,3,4,-1,4]

2. Circular Next Greater – Solution

The following is a solution which demonstrates how to find the next greater element in a circular array.

This solution uses the monotonic stack approach. This solution finds the next greater element for each array value, in the first pass, and then uses a second pass to process any remaining values since the array is circular.





		
		
			2. Circular Next Greater - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 15, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to find the next greater element in a 
//        circular array
// ============================================================================
public class Solution {
    public int[] NextGreaterElements(int[] nums) {
        var result = GetNextGreatest(nums);
        return result;
    }

    public int[] GetNextGreatest(int[] nums) {
        var result = new int[nums.Length];
        var stack = new Stack<int>();

        for (int index = 0; index < nums.Length; ++index) {
            // Default to -1 for this index
            result[index] = -1;

            // Fill in values that have a greater element
            while (stack.Count > 0 && nums[stack.Peek()] < nums[index]) {
                result[stack.Peek()] = nums[index];
                stack.Pop();
            }

            // Add index to stack
            stack.Push(index);
        }

        // Fill in any left over values since this is circular
        for (int index = 0; index < nums.Length && stack.Count > 0; ++index) {
            while (stack.Count > 0 && nums[stack.Peek()] < nums[index]) {
                result[stack.Peek()] = nums[index];
                stack.Pop();
            }
        }

        return result;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 15, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to find the next greater element in a 
//        circular array
// ============================================================================
public class Solution {
    public int[] NextGreaterElements(int[] nums) {
        var result = GetNextGreatest(nums);
        return result;
    }
 
    public int[] GetNextGreatest(int[] nums) {
        var result = new int[nums.Length];
        var stack = new Stack<int>();
 
        for (int index = 0; index < nums.Length; ++index) {
            // Default to -1 for this index
            result[index] = -1;
 
            // Fill in values that have a greater element
            while (stack.Count > 0 && nums[stack.Peek()] < nums[index]) {
                result[stack.Peek()] = nums[index];
                stack.Pop();
            }
 
            // Add index to stack
            stack.Push(index);
        }
 
        // Fill in any left over values since this is circular
        for (int index = 0; index < nums.Length && stack.Count > 0; ++index) {
            while (stack.Count > 0 && nums[stack.Peek()] < nums[index]) {
                result[stack.Peek()] = nums[index];
                stack.Pop();
            }
        }
 
        return result;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[2,-1,2] [2,3,4,-1,4]

C# || How To Find The Next Greater Element In An Array Using C#

October 14, 2021 admin No comments

The following is a module with functions which demonstrates how to find the next greater element in an array using C#.

1. Next Greater – Problem Statement

The next greater element of some element x in an array is the first greater element that is to the right of x in the same array.

You are given two distinct 0-indexed integer arrays nums1 and nums2, where nums1 is a subset of nums2.

For each 0 <= i < nums1.length, find the index j such that nums1[i] == nums2[j] and determine the next greater element of nums2[j] in nums2. If there is no next greater element, then the answer for this query is -1.

Return an array ans of length nums1.length such that ans[i] is the next greater element as described above.

Example 1:

Input: nums1 = [4,1,2], nums2 = [1,3,4,2] Output: [-1,3,-1] Explanation: The next greater element for each value of nums1 is as follows: - 4 is underlined in nums2 = [1,3,4,2]. There is no next greater element, so the answer is -1. - 1 is underlined in nums2 = [1,3,4,2]. The next greater element is 3. - 2 is underlined in nums2 = [1,3,4,2]. There is no next greater element, so the answer is -1.

Example 2:

Input: nums1 = [2,4], nums2 = [1,2,3,4] Output: [3,-1] Explanation: The next greater element for each value of nums1 is as follows: - 2 is underlined in nums2 = [1,2,3,4]. The next greater element is 3. - 4 is underlined in nums2 = [1,2,3,4]. There is no next greater element, so the answer is -1.

2. Next Greater – Solution

The following is a solution which demonstrates how to find the next greater element in an array.

This solution uses the monotonic stack approach. This solution finds the next greater element for each array value in the second array, and uses that to find the next greater element for each matching value in the first array.

To determine the next greatest element, a stack is used to keep track of the items we’ve already seen. The array index of the item is saved to the stack.

For each loop iteration, the item at the top of the stack is checked to see if it is less than the current array item being checked. If the item at the top of the stack is less than the current array item, then the current array item is saved to the result index that matches the value from the top of the stack.

Once the next greatest elements have been found from nums2, that information is used to populate the final result from the matching values found in nums1





		
		
			2. Next Greater - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 14, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to find the next greater element in an array
// ============================================================================
public class Solution {
    public int[] NextGreaterElement(int[] nums1, int[] nums2) {

        // For each element in nums2, get the next greatest element
        var nums2NextGreatest = GetNextGreatest(nums2);

        // Create a dictionary with the next greatest of each element from nums2
        var seen = new Dictionary<int, int>();
        for (int index = 0; index < nums2.Length; ++index) {
            seen[nums2[index]] = nums2NextGreatest[index];
        }

        // From the items in the dictionary, populate the results
        var result = new int[nums1.Length];
        for (int index = 0; index < nums1.Length; ++index) {
            result[index] = seen[nums1[index]];
        }

        return result;
    }

    public int[] GetNextGreatest(int[] nums) {
        // Create a stack that keeps track of the item and its index
        var stack = new Stack<int>();
        var result = new int[nums.Length];

        // Go through array and find the next greatest value
        for (int index = 0; index < nums.Length; ++index) {
            // Default the value at index to -1
            result[index] = -1;

            // Check to see if the item at the top of the stack is less than current item
            while (stack.Count > 0 && nums[stack.Peek()] < nums[index]) {
                // Save the current item at the result index
                result[stack.Peek()] = nums[index];
                stack.Pop();
            }

            // Save array index to the stack
            stack.Push(index);
        }

        return result;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 14, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to find the next greater element in an array
// ============================================================================
public class Solution {
    public int[] NextGreaterElement(int[] nums1, int[] nums2) {
 
        // For each element in nums2, get the next greatest element
        var nums2NextGreatest = GetNextGreatest(nums2);
 
        // Create a dictionary with the next greatest of each element from nums2
        var seen = new Dictionary<int, int>();
        for (int index = 0; index < nums2.Length; ++index) {
            seen[nums2[index]] = nums2NextGreatest[index];
        }
 
        // From the items in the dictionary, populate the results
        var result = new int[nums1.Length];
        for (int index = 0; index < nums1.Length; ++index) {
            result[index] = seen[nums1[index]];
        }
 
        return result;
    }
 
    public int[] GetNextGreatest(int[] nums) {
        // Create a stack that keeps track of the item and its index
        var stack = new Stack<int>();
        var result = new int[nums.Length];
 
        // Go through array and find the next greatest value
        for (int index = 0; index < nums.Length; ++index) {
            // Default the value at index to -1
            result[index] = -1;
 
            // Check to see if the item at the top of the stack is less than current item
            while (stack.Count > 0 && nums[stack.Peek()] < nums[index]) {
                // Save the current item at the result index
                result[stack.Peek()] = nums[index];
                stack.Pop();
            }
 
            // Save array index to the stack
            stack.Push(index);
        }
 
        return result;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[-1,3,-1] [3,-1]

C# || How To Multiply Two Strings Using C#

October 13, 2021 admin No comments

The following is a module with functions which demonstrates how to multiply two strings together using C#.

1. Multiply Strings – Problem Statement

Given two non-negative integers num1 and num2 represented as strings, return the product of num1 and num2, also represented as a string.

Note: You must not use any built-in BigInteger library or convert the inputs to integer directly.

Example 1:

Input: num1 = "2", num2 = "3" Output: "6"

Example 2:

Input: num1 = "123", num2 = "456" Output: "56088"

2. Multiply Strings – Solution

The following is a solution which demonstrates how to multiply two strings together.

This solution starts from the end of both strings and multiplies each individual number, keeping track of any carryovers. The result of each operation is stored in a ‘solution’ array, and when the operation is complete, the result is returned as a string.





		
		
			2. Multiply Strings - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to multiply two strings together
// ============================================================================
public class Solution {
    public string Multiply(string num1, string num2) {
        if (num1 ==  "0" || num2 == "0") {
            return "0";
        }

        // This will be the max number of digits
        var solution = new int[num1.Length + num2.Length];

        // Loop through both strings from the end
        for (int num1Index = num1.Length -1; num1Index >= 0; --num1Index) {
            for (int num2Index = num2.Length -1; num2Index >= 0; --num2Index) {
                // Get the current solution index
                var currentIndex = num1Index + num2Index + 1;

                // Multiply the two numbers
                var value = CharToInt(num1[num1Index]) * CharToInt(num2[num2Index]);

                // Add the value to the result at the current index
                solution[currentIndex] += value;

                // Check to see if there is any carry to be removed
                if (solution[currentIndex] > 9) {

                    // Add the carry to the 'previous' index
                    var carry = solution[currentIndex] / 10;
                    solution[currentIndex - 1] += carry;

                    // Remove the carry from the current index
                    var digit = solution[currentIndex] % 10;
                    solution[currentIndex] = digit;
                }
            }
        }

        // Skip over leading zeroes
        var zeroIndex = 0;
        while (zeroIndex < solution.Length && solution[zeroIndex] == 0) {
            ++zeroIndex;
        }

        // Convert to string and return
        var result = new StringBuilder();
        for (int index = zeroIndex; index < solution.Length; ++index) {
            result.Append(solution[index]);
        }

        return result.ToString();
    }

    public int CharToInt(char ch) {
        return ch - '0';
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to multiply two strings together
// ============================================================================
public class Solution {
    public string Multiply(string num1, string num2) {
        if (num1 ==  "0" || num2 == "0") {
            return "0";
        }
 
        // This will be the max number of digits
        var solution = new int[num1.Length + num2.Length];
 
        // Loop through both strings from the end
        for (int num1Index = num1.Length -1; num1Index >= 0; --num1Index) {
            for (int num2Index = num2.Length -1; num2Index >= 0; --num2Index) {
                // Get the current solution index
                var currentIndex = num1Index + num2Index + 1;
 
                // Multiply the two numbers
                var value = CharToInt(num1[num1Index]) * CharToInt(num2[num2Index]);
 
                // Add the value to the result at the current index
                solution[currentIndex] += value;
 
                // Check to see if there is any carry to be removed
                if (solution[currentIndex] > 9) {
 
                    // Add the carry to the 'previous' index
                    var carry = solution[currentIndex] / 10;
                    solution[currentIndex - 1] += carry;
 
                    // Remove the carry from the current index
                    var digit = solution[currentIndex] % 10;
                    solution[currentIndex] = digit;
                }
            }
        }
 
        // Skip over leading zeroes
        var zeroIndex = 0;
        while (zeroIndex < solution.Length && solution[zeroIndex] == 0) {
            ++zeroIndex;
        }
 
        // Convert to string and return
        var result = new StringBuilder();
        for (int index = zeroIndex; index < solution.Length; ++index) {
            result.Append(solution[index]);
        }
 
        return result.ToString();
    }
 
    public int CharToInt(char ch) {
        return ch - '0';
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

"6" "56088"

C# || How To Add Two Binary Strings Using C#

October 13, 2021 admin No comments

The following is a module with functions which demonstrates how to add two binary strings together using C#.

1. Add Binary – Problem Statement

Given two binary strings a and b, return their sum as a binary string.

Example 1:

Input: a = "11", b = "1" Output: "100"

Example 2:

Input: a = "1010", b = "1011" Output: "10101"

2. Add Binary – Solution

The following is a solution which demonstrates how to add two binary strings together.

In this solution, we start at the end of both strings, and perform basic math on each number, adding them together. If any mathematical carry over is required, that is added to the next loop iteration.





		
		
			2. Add Binary - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to add two binary strings together
// ============================================================================
public class Solution {
    public string AddBinary(string a, string b) {
        // Process numbers starting from the end
        var aLength = a.Length - 1;
        var bLength = b.Length - 1;

        // Go through data and add each number together
        var stack = new Stack<int>();
        var carry = 0;
        while (aLength >= 0 || bLength >= 0) {
            var aValue = 0;
            var bValue = 0;

            if (aLength >= 0) {
                aValue = CharToInt(a[aLength--]);
            }
            if (bLength >= 0) {
                bValue = CharToInt(b[bLength--]);
            }

            // Add the a and b values together, including carry
            var sum = carry + aValue + bValue;

            // Determine the carry over value
            carry = sum / 2;

            // Save the digit
            var digit = sum % 2;
            stack.Push(digit);
        }

        // Add any data left over
        if (carry > 0) {
            stack.Push(carry);
        }

        // Save results
        var result = new StringBuilder();
        while (stack.Count > 0) {
            result.Append(stack.Peek());
            stack.Pop();
        }

        return result.ToString();
    }

    private int CharToInt(char ch) {
        return ch - '0';
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to add two binary strings together
// ============================================================================
public class Solution {
    public string AddBinary(string a, string b) {
        // Process numbers starting from the end
        var aLength = a.Length - 1;
        var bLength = b.Length - 1;
 
        // Go through data and add each number together
        var stack = new Stack<int>();
        var carry = 0;
        while (aLength >= 0 || bLength >= 0) {
            var aValue = 0;
            var bValue = 0;
 
            if (aLength >= 0) {
                aValue = CharToInt(a[aLength--]);
            }
            if (bLength >= 0) {
                bValue = CharToInt(b[bLength--]);
            }
 
            // Add the a and b values together, including carry
            var sum = carry + aValue + bValue;
 
            // Determine the carry over value
            carry = sum / 2;
 
            // Save the digit
            var digit = sum % 2;
            stack.Push(digit);
        }
 
        // Add any data left over
        if (carry > 0) {
            stack.Push(carry);
        }
 
        // Save results
        var result = new StringBuilder();
        while (stack.Count > 0) {
            result.Append(stack.Peek());
            stack.Pop();
        }
 
        return result.ToString();
    }
 
    private int CharToInt(char ch) {
        return ch - '0';
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

"100" "10101"

C# || How To Traverse A Binary Tree Postorder Using C#

October 13, 2021 admin No comments

The following is a module with functions which demonstrates how to traverse a binary tree post order using C#.

1. Binary Tree Traversal – Problem Statement

Given the root of a binary tree, return the postorder traversal of its nodes’ values.

Example 1:

Input: root = [1,null,2,3] Output: [3,2,1]

Example 2:

Input: root = [] Output: []

Example 3:

Input: root = [1] Output: [1]

Example 4:

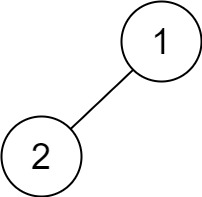
Input: root = [1,2] Output: [2,1]

Example 5:

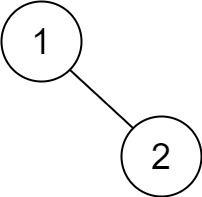
Input: root = [1,null,2] Output: [2,1]

2. Binary Tree Traversal – Solution

The following is a solution which demonstrates how to traverse a binary tree post order.





		
		
			2. Binary Tree Traversal - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to traverse a binary tree post order
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private List<int> result = new List<int>();
    
    public IList<int> PostorderTraversal(TreeNode root) {
        Traverse(root);
        return result;        
    }
    
    public void Traverse(TreeNode node) {
        if (node == null) {
            return;
        }        
        
        Traverse(node.left);
        
        Traverse(node.right);
        
        result.Add(node.val);        
    }    
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to traverse a binary tree post order
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private List<int> result = new List<int>();
    
    public IList<int> PostorderTraversal(TreeNode root) {
        Traverse(root);
        return result;        
    }
    
    public void Traverse(TreeNode node) {
        if (node == null) {
            return;
        }        
        
        Traverse(node.left);
        
        Traverse(node.right);
        
        result.Add(node.val);        
    }    
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[3,2,1] [] [1] [2,1] [2,1]

C# || How To Traverse A Binary Tree Preorder Using C#

October 13, 2021 admin 1 Comment

The following is a module with functions which demonstrates how to traverse a binary tree pre order using C#.

1. Binary Tree Traversal – Problem Statement

Given the root of a binary tree, return the preorder traversal of its nodes’ values.

Example 1:

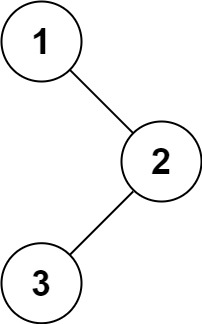
Input: root = [1,null,2,3] Output: [1,2,3]

Example 2:

Input: root = [] Output: []

Example 3:

Input: root = [1] Output: [1]

Example 4:

Input: root = [1,2] Output: [1,2]

Example 5:

Input: root = [1,null,2] Output: [1,2]

2. Binary Tree Traversal – Solution

The following is a solution which demonstrates how to traverse a binary tree pre order.





		
		
			2. Binary Tree Traversal - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to traverse a binary tree pre order
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private List<int> result = new List<int>();
    
    public IList<int> PreorderTraversal(TreeNode root) {
        Traverse(root);
        return result;        
    }
    
    public void Traverse(TreeNode node) {
        if (node == null) {
            return;
        }
        
        result.Add(node.val);
        
        Traverse(node.left);
        
        Traverse(node.right);
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to traverse a binary tree pre order
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private List<int> result = new List<int>();
    
    public IList<int> PreorderTraversal(TreeNode root) {
        Traverse(root);
        return result;        
    }
    
    public void Traverse(TreeNode node) {
        if (node == null) {
            return;
        }
        
        result.Add(node.val);
        
        Traverse(node.left);
        
        Traverse(node.right);
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[1,2,3] [] [1] [1,2] [1,2]

C# || How To Traverse A Binary Tree Inorder Using C#

October 13, 2021 admin No comments

The following is a module with functions which demonstrates how to traverse a binary tree in order using C#.

1. Binary Tree Traversal – Problem Statement

Given the root of a binary tree, return the inorder traversal of its nodes’ values.

Example 1:

Input: root = [1,null,2,3] Output: [1,3,2]

Example 2:

Input: root = [] Output: []

Example 3:

Input: root = [1] Output: [1]

Example 4:

Input: root = [1,2] Output: [2,1]

Example 5:

Input: root = [1,null,2] Output: [1,2]

2. Binary Tree Traversal – Solution

The following is a solution which demonstrates how to traverse a binary tree in order.





		
		
			2. Binary Tree Traversal - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to traverse a binary tree in order
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private List<int> result = new List<int>();

    public IList<int> InorderTraversal(TreeNode root) {
        Traverse(root);
        return result;
    }

    public void Traverse(TreeNode node) {
        if (node == null) {
            return;
        }

        Traverse(node.left);

        result.Add(node.val);

        Traverse(node.right);
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Demonstrates how to traverse a binary tree in order
// ============================================================================
/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     public int val;
 *     public TreeNode left;
 *     public TreeNode right;
 *     public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
public class Solution {
    private List<int> result = new List<int>();
 
    public IList<int> InorderTraversal(TreeNode root) {
        Traverse(root);
        return result;
    }
 
    public void Traverse(TreeNode node) {
        if (node == null) {
            return;
        }
 
        Traverse(node.left);
 
        result.Add(node.val);
 
        Traverse(node.right);
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

[1,3,2] [] [1] [2,1] [1,2]

C# || How To Determine The Maximum Units On A Truck Using C#

October 13, 2021 admin No comments

The following is a module with functions which demonstrates how to determine the maximum units on a truck using C#.

1. Maximum Units – Problem Statement

You are assigned to put some amount of boxes onto one truck. You are given a 2D array boxTypes, where boxTypes[i] = [numberOfBoxes, numberOfUnitsPerBox]:

• numberOfBoxes is the number of boxes of type i. • numberOfUnitsPerBox is the number of units in each box of the type i

You are also given an integer truckSize, which is the maximum number of boxes that can be put on the truck. You can choose any boxes to put on the truck as long as the number of boxes does not exceed truckSize.

Return the maximum total number of units that can be put on the truck.

Example 1:

Input: boxTypes = [[1,3],[2,2],[3,1]], truckSize = 4 Output: 8 Explanation: There are: - 1 box of the first type that contains 3 units. - 2 boxes of the second type that contain 2 units each. - 3 boxes of the third type that contain 1 unit each. You can take all the boxes of the first and second types, and one box of the third type. The total number of units will be = (1 * 3) + (2 * 2) + (1 * 1) = 8.

Example 2:

Input: boxTypes = [[5,10],[2,5],[4,7],[3,9]], truckSize = 10 Output: 91

2. Maximum Units – Solution

The following is a solution which demonstrates how to determine the maximum units on a truck.

In this solution, we start with the box with the most units. The box types are sorted by the number of units per box in descending order. Then, the box types are iterated over, taking from each type as many as possible.





		
		
			2. Maximum Units - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines the maximum units you can put on a truck
// ============================================================================
public class Solution {
    public int MaximumUnits(int[][] boxTypes, int truckSize) {
        var result = 0;

        // Sort the box types by number of units per box in descending order
        Array.Sort(boxTypes, (a, b) => {
           return b[1] - a[1];
        });

        // Determine how many units can be added to the truck
        var remainingSize = truckSize;
        foreach (var type in boxTypes) {
            // Get the box count and units per box
            var boxCount = type[0];
            var unitsPerBox = type[1];

            // Check to see if theres enough room to add more boxes
            var adjustedBoxCount = 0;
            if (remainingSize - boxCount > 0) {
                adjustedBoxCount = boxCount; // All boxes can be added
            } else {
                adjustedBoxCount = remainingSize; // Only the remaining amount can be added
            }

            // Add the units to the result
            result += adjustedBoxCount * unitsPerBox;

            // Adjust remaining truck size with the added boxes
            remainingSize -= adjustedBoxCount;

            // Exit if there is no more room remaining
            if (remainingSize == 0) {
                break;
            }
        }

        return result;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 13, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines the maximum units you can put on a truck
// ============================================================================
public class Solution {
    public int MaximumUnits(int[][] boxTypes, int truckSize) {
        var result = 0;
 
        // Sort the box types by number of units per box in descending order
        Array.Sort(boxTypes, (a, b) => {
           return b[1] - a[1];
        });
 
        // Determine how many units can be added to the truck
        var remainingSize = truckSize;
        foreach (var type in boxTypes) {
            // Get the box count and units per box
            var boxCount = type[0];
            var unitsPerBox = type[1];
 
            // Check to see if theres enough room to add more boxes
            var adjustedBoxCount = 0;
            if (remainingSize - boxCount > 0) {
                adjustedBoxCount = boxCount; // All boxes can be added
            } else {
                adjustedBoxCount = remainingSize; // Only the remaining amount can be added
            }
 
            // Add the units to the result
            result += adjustedBoxCount * unitsPerBox;
 
            // Adjust remaining truck size with the added boxes
            remainingSize -= adjustedBoxCount;
 
            // Exit if there is no more room remaining
            if (remainingSize == 0) {
                break;
            }
        }
 
        return result;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

8 91

C# || Word Search – How To Search A Grid Matrix For A Target Word Using C#

October 11, 2021 admin No comments

The following is a module with functions which demonstrates how to search a grid matrix for a target word using C#.

1. Word Search – Problem Statement

Given an m x n grid of characters board and a string word, return true if word exists in the grid.

The word can be constructed from letters of sequentially adjacent cells, where adjacent cells are horizontally or vertically neighboring. The same letter cell may not be used more than once.

Example 1:

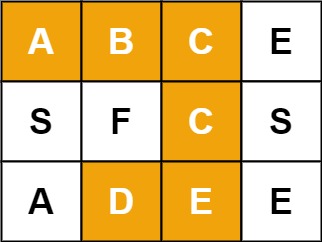
Input: board = [["A","B","C","E"],["S","F","C","S"],["A","D","E","E"]], word = "ABCCED" Output: true

Example 2:

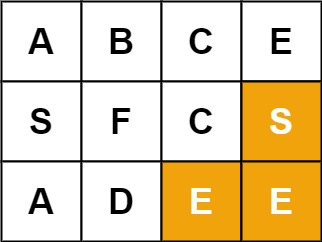
Input: board = [["A","B","C","E"],["S","F","C","S"],["A","D","E","E"]], word = "SEE" Output: true

Example 3:

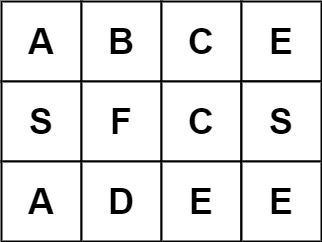
Input: board = [["A","B","C","E"],["S","F","C","S"],["A","D","E","E"]], word = "ABCB" Output: false

2. Word Search – Solution

The following is a solution which demonstrates how to search a grid matrix for a target word. This solution uses Depth First Search.

Depth First Search (DFS) is an algorithm for searching tree or graph data structures. It starts at the root node and explores as far as possible along each branch before backtracking.

In this problem we start at the root node and search all the possibilities at each array index until a match is found. We create a ‘visited’ array to keep track of the indexes already seen.





		
		
			2. Word Search - Solution
			
C#
			
			// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 11, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines if a word can be constructed from letters in a grid
// ============================================================================
public class Solution {
    public bool Exist(char[][] board, string word) {

        // Create a 2D bool 'visited' node matrix to keep track of the
        // items we've already seen
        var rowsVisited = new bool[board.Length][];
        for (int rowIndex = 0; rowIndex < board.Length; ++rowIndex) {
            rowsVisited[rowIndex] = new bool[board[rowIndex].Length];
        }

        // Start at the root node and explore as far as possible along each branch
        for (int rowIndex = 0; rowIndex < board.Length; ++rowIndex) {
            for (int colIndex = 0; colIndex < board[rowIndex].Length; ++colIndex) {
                if (DFS(board, rowIndex, colIndex, 0, word, rowsVisited)) {
                    return true;
                }
            }
        }
        return false;
    }

    private bool DFS(char[][] board, int row, int col, int searchIndex, string word, bool[][] rowsVisited) {
        // This is the success case
        if (searchIndex >= word.Length) {
            return true;            
        }
        // Make sure the search parameters are in bounds
        if (row < 0 || row >= board.Length || col < 0 || col >= board[row].Length) {
            return false;            
        }       
        if (rowsVisited[row][col]) {
            return false;            
        } 
        if (board[row][col] != word[searchIndex]) {
            return false;            
        }        
        
        // Mark that this row has been visited
        rowsVisited[row][col] = true;
        
        var searchResult = 
            // Search left
            DFS(board, row, col - 1, searchIndex + 1, word, rowsVisited) ||
            
            // Search right
            DFS(board, row, col + 1, searchIndex + 1, word, rowsVisited) ||
            
            // Search top
            DFS(board, row - 1, col, searchIndex + 1, word, rowsVisited) ||
            
            // Search bottom
            DFS(board, row + 1, col, searchIndex + 1, word, rowsVisited);
        
        // Unmark that this row has been visited
        rowsVisited[row][col] = false;
        
        return searchResult;
    }
}// http://programmingnotes.org/
			
				
					
				
					1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
				
						// ============================================================================
//    Author: Kenneth Perkins
//    Date:   Oct 11, 2021
//    Taken From: http://programmingnotes.org/
//    File:  Solution.cs
//    Description: Determines if a word can be constructed from letters in a grid
// ============================================================================
public class Solution {
    public bool Exist(char[][] board, string word) {
 
        // Create a 2D bool 'visited' node matrix to keep track of the
        // items we've already seen
        var rowsVisited = new bool[board.Length][];
        for (int rowIndex = 0; rowIndex < board.Length; ++rowIndex) {
            rowsVisited[rowIndex] = new bool[board[rowIndex].Length];
        }
 
        // Start at the root node and explore as far as possible along each branch
        for (int rowIndex = 0; rowIndex < board.Length; ++rowIndex) {
            for (int colIndex = 0; colIndex < board[rowIndex].Length; ++colIndex) {
                if (DFS(board, rowIndex, colIndex, 0, word, rowsVisited)) {
                    return true;
                }
            }
        }
        return false;
    }
 
    private bool DFS(char[][] board, int row, int col, int searchIndex, string word, bool[][] rowsVisited) {
        // This is the success case
        if (searchIndex >= word.Length) {
            return true;            
        }
        // Make sure the search parameters are in bounds
        if (row < 0 || row >= board.Length || col < 0 || col >= board[row].Length) {
            return false;            
        }       
        if (rowsVisited[row][col]) {
            return false;            
        } 
        if (board[row][col] != word[searchIndex]) {
            return false;            
        }        
        
        // Mark that this row has been visited
        rowsVisited[row][col] = true;
        
        var searchResult = 
            // Search left
            DFS(board, row, col - 1, searchIndex + 1, word, rowsVisited) ||
            
            // Search right
            DFS(board, row, col + 1, searchIndex + 1, word, rowsVisited) ||
            
            // Search top
            DFS(board, row - 1, col, searchIndex + 1, word, rowsVisited) ||
            
            // Search bottom
            DFS(board, row + 1, col, searchIndex + 1, word, rowsVisited);
        
        // Unmark that this row has been visited
        rowsVisited[row][col] = false;
        
        return searchResult;
    }
}// http://programmingnotes.org/

QUICK NOTES:
The highlighted lines are sections of interest to look out for.

The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.

Once compiled, you should get this as your output for the example cases:

true true false

Monthly Archives: October 2021

C# || How To Determine If Binary Tree Root To Leaf Path Sum Exists Using C#

C# || How To Convert Sorted Array To Binary Search Tree Using C#

C# || How To Convert 1D Array Into 2D Array Using C#

C# || How To Find Cousins In A Binary Tree Using C#

C# || How To Construct A Binary Search Tree From Preorder Traversal Using C#

C# || How To Find The Next Greater Element In A Circular Array Using C#

C# || How To Find The Next Greater Element In An Array Using C#

C# || How To Multiply Two Strings Using C#

C# || How To Add Two Binary Strings Using C#

C# || How To Traverse A Binary Tree Postorder Using C#

C# || How To Traverse A Binary Tree Preorder Using C#

C# || How To Traverse A Binary Tree Inorder Using C#

C# || How To Determine The Maximum Units On A Truck Using C#

C# || Word Search – How To Search A Grid Matrix For A Target Word Using C#

Search

Compile Your Code Now

Translate Page

Popular Pages

Recent

Archives

Categories